Class Activities on Perspective Drawing and Projective Geometry
The purpose of the lab component of the course
is to extend and build connections to course material
in an interactive manner. During labs you will work at your own pace,
preferably in groups of 2-3, as I make my way around the room to check in
(and help!).
Read and complete the activities as you answer the following questions on
a sheet of paper.
- First take out your homework notes and get started below.
Show your hw to me as I make my way around
the room and ask me any questions--I'm happy to help!
Activity 1: Come One - Come All - to a Better Cube
A picture drawn in perspective
was drawn assuming that it would be viewed with one eye from a distance
d behind V, and so this is the mathematically
optimal viewing place and distance.
To test out our determination of d,
Click on
this link of the large drawing
and do as follows as you have a partner read you these directions:
- Use a thumb and a forefinger to measure the distance d between
V and V' which you should see as 2 dots at the very top of your screen.
Keep this distance, called d, with your hand.
- Continuing to keep that distance, turn your hand
to measure out d units in front of V (so d is now
perpendicular to the screen instead of being inside of it).
- Place your left eye exactly at that same point (d
units in front of V -
not in the center of the page). You will need to get very close
to the computer screen to do this.
- Close your right eye.
- Without changing your position, let your left eye roll down and to the
left and then look at the box. Although it may be too close for
comfortable viewing because you will be so close to the
computer screen, the distortion should go away
and it should look much more like a cube! Most people will be able to
see this by following the directions, but some people may have problems
due to astigmatism...
- Now switch roles and
read the directions to your partner as they complete this activity.
- Were you able to see the distortion disappear?
- As a review, briefly summarize where was your left eye placed?
Viewers in a gallery or museum almost never
assume the correct perspective viewpoint, because these locations tend to be
rather low, off-center, rather close, etc. It's true that the artwork may
appear perfectly acceptable from most locations in the room. However, when
one assumes the correct viewpoint and looks with one eye, it is common for
the illusion of depth to be astonishingly more believable. You can almost
"feel" the space!
(Of course the work must be of high quality and very precise.)
While you can't draw dashed lines on the artwork to find the viewing
distance, you can mentally trace appropriate lines by holding up shish
kebab skewers. Once you do so, you can use your hands to measure the
viewing distance in the painting and stand d units in front of V.


Activity 2: Using Mathematics to Create Precise Perspective Drawings and Computer Animations
-
Given a point (x,y,z) of a real-life object with d > 0,
write out the mathematical formulas for the
perspective drawing coordinates
of x' and y' that were given on
the homework reading.
We are going to make the computer create a perspective drawing of a house
by using these equations. Work with a partner - have one person
read the directions on their screen while the other performs the
instructions.
Microsoft Excel is an electronic spreadsheet program that we'll use to
algebraically manipulate and analyze data and create visualizations. Data
is organized into boxes or cells that are labeled by their row number and
column letter.
Download and open this
Excel file using the program with the green X.
You will see a chart that is partly filled in with
real-life x, y and z
coordinates of a house (in columns A, B and C, respectively).
We will use the viewing distance of 15 (as in column D)
to calculate x' and y', and create a perspective drawing of it in Excel.
So, we want to mathematically project
the three dimensional house onto the
mathematically precise perspective image in the plane (where we can draw it).
So, we want to transform x, y and z to new coordinates
x'=(d x)/(z+d) and y'=(d y) /(z+d).
We will make Excel do these formulas for us!
- To transform x to x', click on E2 in the Excel sheet
(row 2, column E).
The Excel version of the formula for x' that you should type in is:
=d2*a2/(c2+d2)
so type this formula (including the equals sign)
into E2 and hit return. You should now see -1.875.
Make sure you understand the above before you continue.
To fill down the formula to the other rows, we'll force Excel to change the
2s to 3s to 4s and so on.
Click on E2 again.
At the bottom right corner of E2 scroll until you get a square
with arrows which looks like  .
Then click, hold down, and
fill down the Excel column by scrolling down and releasing in E18. The number
you will see there is -2.7631579.
.
Then click, hold down, and
fill down the Excel column by scrolling down and releasing in E18. The number
you will see there is -2.7631579.
- What Excel formula should we use in F2
corresponding to y'=(d y)/(z+d) (for the second row)?
Enter your formula into F2 and hit return. You should now see -2.8125.
At the bottom corner of F2 click until you get a square with
arrows. Then fill down the Excel column and release in F18. The number
you will see there is .39473684.
- To draw our house, click on the grey E box, so that that column
is highlighted.
Then hold down the command key while you click on the
grey F box, so that both the E and F columns are now highlighted.
Under the Insert menu, release on Chart
and then Scatter or XY (Scatter).
Now we have our mathematical drawing, but it doesn't
look very impressive. Some of the points of the house are behind our
viewpoint, and are only there if we want to change our view (like by making
the house rotate).
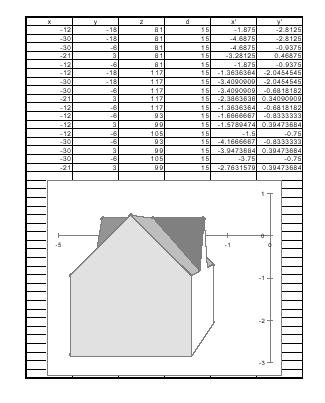
To see the final picture, all we would have
to do is connect the dots and shade in the figure,
as in the image below (but don't do this now)
Digital Movies
Digital animations such as
 use many more rows of Excel. The full-body version of
this Yoda uses 53,756 vertices!
use many more rows of Excel. The full-body version of
this Yoda uses 53,756 vertices!
Models created by Kecskemeti B. Zoltan and visualized by T. Chartier. Images courtesy of Lucasfilm LTD as on
Using the Force of Math in Star Wars
- List the artists and mathematicians who are mentioned in the
perspective drawing homework reading
and give a very brief summary of their contributions.
Example: Julian Beever, pavement drawings in perspective
- Take the
ASULearn Mathematical Experiences reflection
- Look over the hw for tomorrow from the main calendar page and ask
me any questions before you leave (as time allows).
Adapted
from Marc Frantz's Mathematics and Art.


 .
Then click, hold down, and
fill down the Excel column by scrolling down and releasing in E18. The number
you will see there is -2.7631579.
.
Then click, hold down, and
fill down the Excel column by scrolling down and releasing in E18. The number
you will see there is -2.7631579.

 use many more rows of Excel. The full-body version of
this Yoda uses 53,756 vertices!
use many more rows of Excel. The full-body version of
this Yoda uses 53,756 vertices!