| By gluing together corresponding sides of a bowed-in
hyperbolic
version of this
18-sided figure (for example, the pentagon faces get glued
together), we
obtain a
hyperbolic universe. Mathematical objects are sometimes, but not always,
named after the first person to discover them.
Just as Pythagoras was not the first to come up with the
Pythagorean theorem [which goes back to Babylonian times], I was not the
first to come up with the spherical version now known as the Greenwaldian
theorem in the Futurama universe [which probably goes back to
Menelaus of Alexandria]. However, this space known as the Weeks manifold,
after Jeff Weeks, was first discovered by him.
|
Question 10 Each patient is a different row. How many patients were
studied? Hint: subtract 1 from the rows used, since the first row contains the data descriptors rather than a person.
This data was the real data that was used to study patients
in this higher dimensional data space (description of the data). Data was
collected from patients in the US, Hungary and Switzerland.
New patients have since been diagnosed inside of
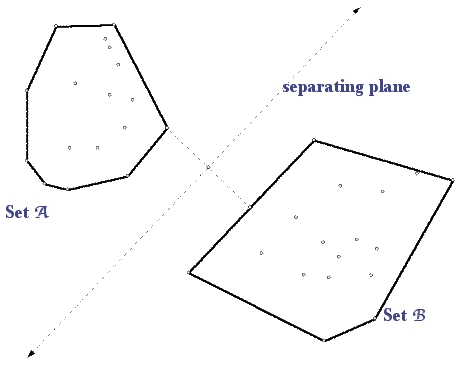
higher dimensions using a higher dimensional separating plane:
|
For example, here experts collect data for a large sample of individuals
and assigned the participants in one of two classes.
Each individual corresponds to a point in an n-dimensional space
where n is the number of measurements recorded for each individual.
Mathematics is then used to
separate the classes via a plane, similar to the idea of linear
regression (which we'll see later on this semester),
but instead of finding a "best fit" line to all of the data,
we find the higher dimensional plane that best separates the data into classes.
| 
|
New individuals are then classified and diagnosed
by a computer using
the separating plane.
Breast Cancer
When a tumor is found, it is important to
diagnose whether it is benign or cancerous.
In real-life,
9 attributes were
obtained via needle aspiration of a tumor such as clump thickness,
uniformity of cell size, and uniformity of cell shape.
The Wisconsin Breast Cancer Database used the data of
682 patients whose cancer status was known.
Since 9 attributes were measured, the data was contained in a
space that had 9 physical dimensions.
A separating plane was obtained.
There has been 100% correctness on computer diagnosis of 131 new
(initially unknown) cases, so this method has been very successful.
You may now quit Excel without saving your file.
What is the 4th Physical Dimension?
We have heard that physicists think that the universe has many
more physical dimensions than we directly experience.
We can try and understand the 4th physical dimension by thinking about
how a 2D Marge can understand the 3rd physical dimension.
For example, when Homer disappears behind the bookcase,
or when she sees shadows of a rotating cube, she experiences
behavior that does not seem to make sense to her.
In fact, since it is 3D behavior, it does not make sense in 2D.
But, it is in this
indirect way that 2D Marge can gain an appreciation for 3D.
Similarly, we can use indirect ways
of trying to gain an appreciation
for the counterintuitive behavior of 4D objects.