Project 6
Problem 1
Part A:
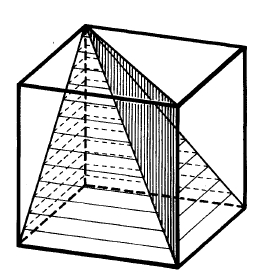
Use this figure (a square pyramid inside a cube)
and Geoblocks (you can sign a kit out from the math/science education
center on the second floor) or models that you build
to give a plausible explanation of why the
volume of a square pyramid is one-third the height times the base.
|
|
Part B: Can you find a similar decomposition
relating a rectangular box and a rectangular pyramid?
Explain.
Part C:
Can you find a similar decomposition relating
a rectangular box and a triangular prism? Explain.
For the following problems,
you may do by-hand work and/or use Sketchpad.
Be sure to show work and
explain (if you use Sketchpad then print out your Sketchpad work
and explain it).
Problem 2
Look at the triangle whose vertices are A=(2,2),
B=(-1,1), and C=(1,-1).
Part A: Show that this triangle is equilateral under the taxicab
metric.
Part B: Is the triangle equilateral or isosceles under the Euclidean
metric?
Problem 3 In order to show that the Pythagorean Theorem
sometimes but not always holds in taxicab geometry...
Part A: Look at the triangle whose vertices are
A=(-3,9), B=(12,4) and C=(0,0). Notice that the slope of AC = -3
while the slope of BC = 1/3 and so AC is perpendicular to BC. Hence this
is a right triangle. Let c be the hypotenuse (opposite vertex C)
and let a be the side opposite vertex A and
b be the side opposite vertex B.
Compute a, b and c under the taxicab metric. Compare a2 +
b2
with c2. Does the Pythagorean theorem hold for this right triangle
in taxicab geometry?
Part B: Look at the triangle whose vertices are
A=(0,0), B=(4,3) and C=(4,0). Notice that this is a
right triangle.
Compare a2 + b2
with c2.
Does the Pythagorean theorem hold for this right triangle in taxicab geometry?
Problem 4
A man has a newspaper stand at W=(1,0), eats regularly at a cafeteria
located at E=(8,3), and does his laundry at a laundromat at
L=(7,2)
Part A:
If he wants to find a room R using the taxicab metric so as to be
at the same walking distance from each of these points, where should R
be located? (Give the coordinates).
Part B:
How many blocks does he have to walk from his room to each of the three points,
assuming that he finds such a room?
Part C:
If all conceivable shortcuts are possible (ie, using the Euclidean metric),
where should the man's room be so that it is equidistant? (Hint:
use the perpendicular bisectors).
Part D:
What is the Euclidean measurement of the distance that
he has to walk from his room in Part C to each of the
three points?
