Euclid Book 1 Proposition 1: To construct an equilateral triangle on a given finite straight line.

Tools

Arrowhead      
Point              
Compass          
Straight Edge      
Alphabet      
Script View      


Sketchpad Construction

  • Choose the Straight Edge Tool and create a segment.
  • Choose the Alphabet Tool and label the endpoints of the line A and B.
  • Choose the Compass Tool, click on A, drag the circle with your mouse and then click so that B lies on the edge of the circle centered at A.
  • Choose the Compass Tool, click on B, drag the circle with your mouse and then click so that A lies on the edge of the circle centered at B.
  • Choose the Point Tool and click down on one of the intersection points of the two circles.
  • Use the Alphabet Tool to label this intersection point as C.
  • Choose the Straight Edge Tool and create the segment AC.
  • Choose the Straight Edge Tool and create the segment BC.
  • Use the Arrowhead Tool to select line AB (make sure that everything else is de-selected first). Under Measure, release on Length.
  • Repeat to measure the lengths of AC and BC.
  • Move points A and B around to see that we still have an equilateral triangle.

    Notes:
              To de-select an object, choose the Arrowhead Tool and click on the white background until the object is no longer highlighted.
              To save your work, under File, release on Save As... and save the file as anyname.gsp (for geometer's sketchpad).
              To create a script view of your work, select all of your work so that it is highlighted via Edit, Select All, and then choose the Script View Tool and release on Create New Tool. Check the Show Script View box and hit ok. To print a script view, Right-click (Windows) or Ctrl-click (Macintosh) on any object in the script, and choose Print Script View from the Context menu that appears:



    2-Column Proof of Proposition 1 - (Euclid's Definitions, Common Notions, Postulates and Propositions can be found in Appendix A (p. 287-292) of Sibley)

    Given segment AB, we will prove that we can construct an equilateral triangle on a given finite straight line.

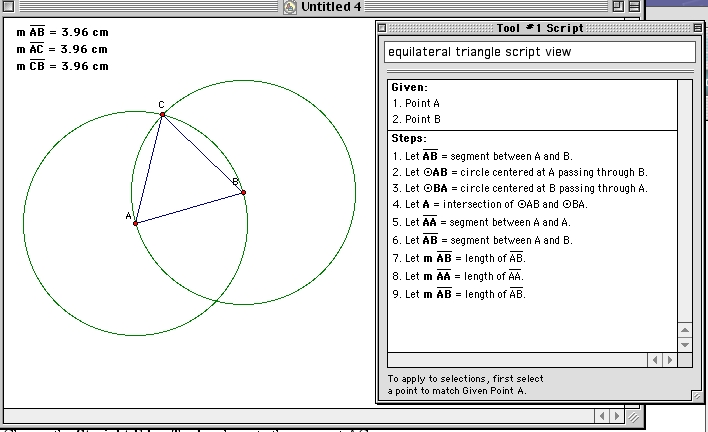
    Draw circle with center A and radius B. Postulate 3
    Draw circle with center B and radius A. Postulate 3
    Let C be an intersection of these circles. Implicit assumption that 2 overlapping circles in the same plane intersect (or need a postulate like If the sum of the radii of two circles is greater than the line joining their centers, then the two circles intersect like on https://mathcs.clarku.edu/~djoyce/java/elements/bookI/propI1.html).
    Connect AC. Postulate 1
    Connect BC.Postulate 1
    Notice that AC=AB. Definition 15 and the fact that AC and AB are radii of the circle with center A.
    Notice that BC=BA. Definition 15 and the fact that BC and BA are radii of the circle with center B.
    Hence AC=AB=BC. Common Notion 1 and the fact that AC=AB and BC=AB.
    Therefore, ABC is equilateral. Definition 20.


    Hence we have constructed an equilateral triangle on a given finite straight line and so we have proven proposition 1.