1. Different parameterizations can lead to very different surfaces
   1. true and I can think of examples
   2. true but I'm not sure why
   3. false but I'm not sure why not
   4. false and I have a reason why not
   
   
   
   
2. The following surfaces were not embedded isometrically via a C² mapping into R³:
   1. models of hyperbolic geometry
   2. stereographic projection of the sphere
   3. flat torus
   4. all of the above
   5. none of the above
   
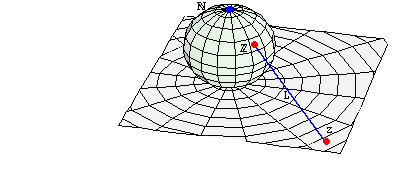
   upper half-plane model       conformal, circles and angles
   
   
   
   
3. Gauss curvature K is
   1. intrinsic
   2. extrinsic
   3. a vector
   4. both a and c
   5. both b and c
   
   
   
   
   
4. Gauss-Bonnet relates
   1. total Gauss curvature in geometry to Euler characteristic in combinatorial topology
   2. Gauss to Bonnet
   3. both of the above
   4. none of the above
   
   
   
   
   
5. I can intuit the principal normal curvatures at a point on a surface
   1. agree
   2. unsure
   3. need help