Problem Set 2

You may work in a group of two and turn in one project per group if you like.

Problem 1

Use the following from Sibley's Geometric Viewpoint to help you, but write out a complete proof that SAS does not hold in R² with the taxicab metric.

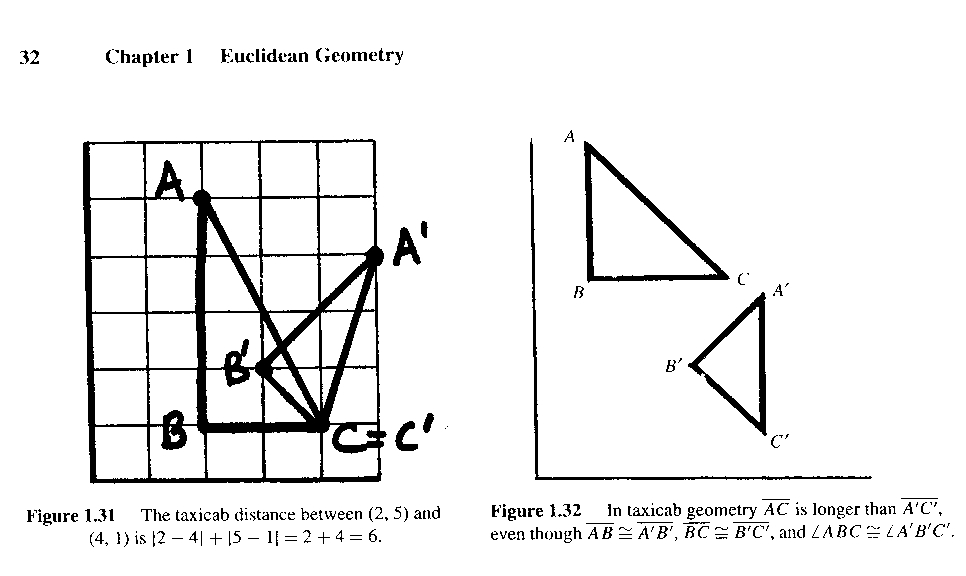
Problem 2

Let (X,d) be a metric space, x in X and Epsilon > 0. Prove that B_d (x, Epsilon) is an open set.

Problem 3

Let (X,d) and (Y,p) be metric spaces. Assume that f: X --> Y is an isometry, ie that f preserves distances. Prove that f is continuous.

Problem 4

Mendelson p. 34 # 3

Problem 5

Assume that X is a set with topologies T1 and T2 on X. Is the union of the two topologies necessarily a topology on X? If so prove the statement, but if not, give a counterexample.

Grad Problems

Grad Problem 6: Mendelson p. 34 # 2

Grad Problem 7: Prove that the intersection of a collection of topologies on X (possibly infinite collection) is still a topology on X.

Extra Credit Problems

Mendelson p. 35 #8,

What is the ball about (0,1) of radius pi in the upper half plane model of hyperbolic space. Justify your answer.

Giving Proper References

Be sure to give proper reference were it is due. Even if you find ideas or the proof elsewhere, all work needs to be written up in your own (group's) words and explanations, although you should still reference the original work or person.