One name that is sure to be near the top of any list of prominent African American mathematicians is that of David Harold Blackwell, Professor Emeritus of Statistics at the University of California at Berkeley. Professor Blackwell�s inclusion on such a list could be for many reasons: He has 80 research publications, 50 Ph.D. students, a reputation for excellent teaching, and an important role as a pioneer in the integration of African Americans into higher mathe-matics. He is also the only African American mathematician elected to membership in the National Academy of Sciences, or to be President or Vice President of the American Statistical Society. Yet, perhaps because he is still alive, resources containing classroom activity sheets on minorities in mathematics do not include him. This is unfortunate since students relate to Blackwell's openness about racial issues and his love of mathematics. In addition, Blackwell has done work in several fields such as game theory and statistical decisions so ideas related to his mathematics can easily be incorporated into various levels of classes.

David Blackwell was born in Centralia, Illinois on April 24, 1919. Early in life he seems to have been spared much of the racial prejudice of early twentieth-century America. While there were segregated elementary schools in Centralia during Blackwell�s youth, one for whites and one for blacks, he attended a school that was integrated. Blackwell describes:

Southern Illinois was probably fairly racist even when I was growing up there. � But I was not even aware of these problems � I had no sense of being discriminated against. My parents protected us from it, and I didn�t encounter enough of it in the schools to notice it.

(Albers & Alexanderson, 1985, p. 19)

Blackwell�s reaction to mathematics in grade school was mixed. While he was not very excited by algebra and trigonometry, he did enjoy geometry. In addition, even at this age Blackwell was intrigued by games such as checkers and naughts and crosses, wondering if there was a strategy so that the player making the first move could always win. He was also a member of the mathematics club in high school. The advisor of the club would challenge the club members with problems from the School Science and Mathematics journal and would submit their solutions. Blackwell was identified in the journal numerous times and one of his solutions was published.

After graduating from high school at the early age of sixteen Blackwell entered the University of Illinois in 1935 planning to be an elementary school teacher. A course on real analysis turned Blackwell to a career in mathematics. �That�s the first time I knew that serious mathematics was for me. It became clear that it was not simply a few things that I liked. The whole subject was just beautiful� (Albers & Alexanderson, 1985, p. 21). At the age of 22, Blackwell received his Ph.D. from Illinois. It was only the seventh Ph.D. in mathematics ever awarded to an African American (Williams, 2002).

Upon graduation, when Blackwell received a one-year appointment as a Rosenwald Postdoctoral Fellow at the prestigious Institute for Advanced Study in Princeton, racism reared its ugly head. It is standard practice for fellows at the Institute for Advanced Study to receive appointments as honorary faculty members at Princeton University. However, in 1941 Princeton had never even had an African American student, much less an African American faculty member, and this produced opposition within the university. The president of the university wrote to the director of the Institute for Advanced Study saying that the Institute was abusing the hospitality of the university with such an appointment. Fortunately, Blackwell was again protected from racial tension, this time by colleagues: �Apparently there was quite a fuss over this, but I didn�t hear a word about it� (Albers & Alexanderson, 1985, p. 23).

During his year in Princeton, Blackwell searched for academic appointments by sending letters only to the over 100 black colleges in the country. He did interview with Jerzy Neyman for a mathematics position at the University of California at Berkeley but no offer was made. Blackwell suggests that

[Racial discrimination] never bothered me. I�ll put it that way. It surely shaped my expectations from the very beginning. It never occurred to me to think about teaching in a major university since it wasn't in my horizon at all.

(DeGroot, 1986, p. 41)

Blackwell received an offer from Southern University in Baton Rouge for an instructorship, and after a year there and a year at Clark University in Atlanta he received a permanent appointment at Howard University. Howard University, by Blackwell�s own admission, �was the ambition of every black scholar. That was the best job you could hope for� (DeGroot, 1986, p. 41). He quickly moved up the ranks, being promoted to full professor in 1947 and serving as chairman of the math department from 1947 until 1954.

In 1945 Blackwell heard Abe Girshick give a lecture on sequential analysis. He later contacted Girshick with what he thought was a counterexample to a theorem presented in the lecture. While Blackwell was incorrect about his counterexample, this contact began a fruitful collaboration between the two men that included their 1954 book Theory of Games and Statistical Decisions. Girshick�s lecture also marked the beginning of Blackwell�s work in statistics. His first publication in the area came a year later.

Blackwell revived his high school interest in the theory of games during his summer employment at Rand Corporation during the summers of 1948-50. While working at Rand, Blackwell became interested in the theory of duels. In the basic game, he first looked at two duelists who start a fixed distance apart with each having a single bullet. When the dual commences, they walk towards each other at a fixed rate. The closer each duelist gets to his opponent before firing, the more likely he is to hit him. The goal is to determine how long to wait before firing. To analyze this problem, the expected value of the dual must be calculated. This situation and other variations on this game are covered in Activity Sheet 1, which is printed on pages 15 through 17 in this issue. Blackwell�s thoughts about another game, called the Prisoner�s Dilemma, are explored in Activity Sheet 2, on page 18.

The culmination of Blackwell�s career at Howard occurred in 1954 when he gave an invited address in probability at the International Congress of Mathematicians in Amsterdam. Shortly after this, perhaps because of changing attitudes towards African Americans in our country, he finally received an appointment at the University of California at Berkeley in the newly formed Department of Statistics. While at Berkeley, Blackwell served as chair of the Statistics Department from 1956 to 1961. He was awarded several honorary doctorates and other awards and distinctions before he retired from the university in 1989. Even after his retirement he has remained active and continued to publish in mathematical journals.

The focuses of Blackwell�s research have been as varied as the universities at which he has taught. His results have applications in economics and accounting, and in 1979 he won the John Von Neumann prize for his work in operations research. However, in an interview with Don Albers (Albers & Alexanderson, 1985, p. 24) Blackwell was asked, �Of the areas in which you have worked, which do you think are most significant?� He replied, �I�ve worked in so many areas: I�m sort of a dilettante. Basically, I�m not interested in doing research and never have been � I�m interested in understanding, which is quite a different thing.� If only we all could understand so much.

References

Albers, D. & Alexanderson, G. (1985). David Blackwell. In D. Albers & G. Alexanderson (Eds.), Mathematical People: Profiles and Interviews (pp. 19-32). Boston: Birkhauser. Condensed version [On-line]. Available: http://scidiv.bcc.ctc.edu/Math/Blackwell.html

DeGroot, M. (1986). A Conversation with David Blackwell. Statistical Science, 1, 40-53.

Houston, J. (1994). David Harold Blackwell - NAM newsletter [On-line]. Available: http://www.maa. org/summa/archive/blackwl.htm

O�Connor, J.J. & Robertson, E.F. (2002) David Harold Blackwell - MacTutor History of Mathematics Archive [On-line]. Available: http://www-gap.dcs.st-and.ac.uk/~history/ Mathematicians/Blackwell.html

Williams, S. (2002). David Blackwell - Mathematicians of the African Diaspora [On-line]. Available: http://www.math.buffalo.edu/ mad /PEEPS/blackwell_david.html

Young, R. (1998). David Blackwell. In Notable Mathematicians : From Ancient Times to the Present (pp. 62-64). Detroit: Gale Research.

Activity Sheet 1: David Blackwell and the Theory of Duels

David Blackwell is cited as one of the pioneers in the theory of duels. He describes how he and his colleagues became interested in duels while working for the Rand Corporation in the late 1940�s:

One day some of us were talking and this question arose: If two people were advancing on each other and each one has a gun with one bullet, when should you shoot? If you miss, you�re required to continue advancing. That�s what gives it dramatic interest. If you fire too early your accuracy is less and there�s a greater chance of missing. It took us about a day to develop the theory of that duel� Then I got the idea of making each gun silent. With the guns silent, if you fire, the other fellow doesn�t know, unless he�s been hit. He doesn�t know whether you fired and missed or whether you still have the bullet. That turned out to be a very interesting problem mathematically.

(Albers & Alexanderson, 1985, p. 25)

His research, which he completed with various coauthors, was among the first rigorous analysis of the age-old concept of a duel, a concept that had many applications in the Cold War era. In this activity sheet, we will explore a simplified version of his work.

In the classic pistol duel, the two duelists start back to back. On command they march a prescribed number of paces away from each other, then turn to face each other, pistols at their sides. Then they raise their smooth bore pistols and each fire a single bullet at the other. If one duelist hits the other, he is considered the winner, while if both are hit or both are missed the duel is considered a draw. It seems to make sense that firing your pistol quickly might be advantageous as you could hit your combatant before he fires and hence throw off his shot. On the other hand, if you wait longer (and presumably aim more accurately) you stand a better chance of hitting your opponent. If you wait long enough to see that your opponent fired his weapon, and you are still standing, you could take as long as you wanted to line up your shot.

We will simulate two simplified versions of this dual. The first will be a �noisy duel� where each duelist can tell when his opponent fires his weapon, and the second a �silent duel� where this information is not available. For both of these games we will make the following assumptions:

The duelists must fire their weapons after either 1,2,3,4,5, or 6 seconds.
If a duelist fires after second i, the probability of hitting their opponent is i/6.
In the noisy duel, if the duelists do not fire their guns at the same time, the duelist who fires second will wait until after 6 seconds thus guaranteeing that he hits his opponent.

Game 1: The Noisy Duel

To simulate this game we need two duelists with a piece of paper and a pair of dice. Before the game starts, each duelist writes down a number from 1 to 6 representing when he plans to fire his weapon. The two numbers are then compared. If the numbers are not equal, the player with the lower number will roll his die to simulate firing his weapon. If his number is i and he rolls 1,2,�,i then he hits his target and wins the duel. If he misses, with a roll of i+1,�6, then the other player will win as he will wait until after 6 seconds to fire and will be guaranteed a hit. If both players picked the same number, they both roll their die to simulate firing their weapon and determine the outcome in the same manner.

Activity 1: Below are some strategies represented as ordered pairs. The pair (i,j) represents Player 1 planning to fire after i seconds and Player 2 planning to fire after j seconds. Below each ordered pair is one or two die rolls. In each case, determine the outcome of the duel.

Strategy	(2,4)	(5,1)	(4,4)	(1,6)	(3,5)	(1,6)
Roll	3	1	3,1	5	3	2
Winner

Activity 2: Now get some dice and find a partner to challenge to a duel. Simulate 10 or 15 duels, and try to find a winning strategy. Hypothesize what you think the optimal strategy might be.

Game 2: The Silent Duel

This game is simulated in the same manner as the noisy duel unless the player who fires first misses. In this case, the other player does not know he has fired and will continue to fire his weapon as planned. Hence two rolls are always needed, and it becomes more likely that both players will miss.

Activity 3: Analyze the strategies and rolls given below and see if you can determine the outcome of each duel. The roll on the left is always Player 1�s roll and the roll on the right is Player 2�s roll, regardless of the order in which they fired.

Strategy	(2,4)	(5,1)	(4,4)	(1,6)	(3,5)	(1,6)
Roll	3,3	1,3	3,1	5,2	3,6	2,3
Winner

Activity 4: Get your dice and partner and prepare to duel. Try to hypothesize an optimal strategy as you simulate 10 or 15 duels.

Mathematical Analysis of the Duel

To analyze these simplified duels we must use some probability theory. In particular, we must look at the expected value of each strategy. To do this we assign a numerical value to each possible outcome of the game. For simplicity we will assign a weight of 1 to a duel in which Player 1 wins and a weight of �1 to a duel in which he loses. A draw will be assigned a value of 0. Now to determine the expected value of each strategy we simply take the sum of each possible outcome times its probability of occurring. One way to think about the expected value of a strategy is that it is the average of the outcomes if this strategy is employed a large number of times. In a duel this may be of limited use since losing once can be quite disastrous.

Since our outcomes are based on a roll of the dice, these probabilities are easy to compute. In real life the calculation may be far more difficult. Also notice that we don�t need to compute the probability of a draw in each strategy as this will be multiplied by the value of a draw: 0. This will greatly simplify our computations.

Game 1: The Noisy Duel

Let�s look at the expected value of the strategy (3,2) in a noisy duel. If this strategy is employed, Player 2 will fire his weapon after 2 seconds. If he hits Player 1, which happens with probability 2/6, Player 1 loses and the duel has a value of �1. If Player 2 misses, which happens with probability 4/6, Player 1 waits for 6 seconds and hits Player 2 with probability 1. In this case the game has a value of 1. Hence the expected value of this strategy is: .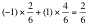

Activity 5: Compute the expected values of the following strategies; (2,5), (5,1), (2,2), (3,3), (3,4), and (3,2) in the noisy duel. The entry in the ith row and jth column of the table given below is the expected outcome of strategy (i,j).

j i	1	2	3	4	5	6
1	0	-4/6	-4/6	-4/6	-4/6	-4/6
2	4/6	0	-2/6	-2/6	-2/6	-2/6
3	4/6	2/6	0	0	0	0
4	4/6	2/6	0	0	2/6	2/6
5	4/6	2/6	0	-2/6	0	4/6
6	4/6	2/6	0	-2/6	-4/6	0

If we are Player 1 and we wish to live through our duel, we want to choose as our strategy the row that has the largest minimum value. This strategy minimizes our chances of losing no matter what strategy Player 2 adapts. Obviously rows 3 or 4 maximize the minimum at 0, that is no matter what Player 2 does, if Player 1 picks strategy 3 or 4 he has at least as good a chance of winning as losing. One could even make the argument that strategy 4 is better than strategy 3 as our chances of winning against strategy 5 or 6 (should Player 2 be foolish enough to choose either of those strategies) would be greater than with strategy 3. This may seem counterintuitive as it says that in a duel where missing before you opponent fires guarantees losing, waiting until you have better than a 50% chance of success is a better strategy.

Game 2: The Silent Duel

The main difference in the analysis of the silent duel is that if the first person misses his shot, this does not guarantee his death. If we look at the expected outcome of strategy (3,2) again we see that Player 2 shoots first and hits Player 1 with probability 2/6. If he misses, which happens with probability 4/6, Player 1 still has to hit his shot, which happens with probability 3/6. Thus the expected value of this strategy is 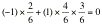. So we see immediately that the silent duel does indeed have different outcomes from the noisy duel.

Activity 6: Compute the expected values of the following strategies; (2,5), (5,1), (2,2), (3,3), (3,4), and (3,2) in the silent duel. The table below gives the expected value for all of the strategies for the silent duel. The entry in the ith row jth column gives the value for strategy (i,j).

j i	1	2	3	4	5	6
1	0	-4/36	-9/36	-14/36	-19/36	-24/36
2	4/36	0	0	-4/36	-8/36	-12/36
3	9/36	0	0	6/36	3/36	0
4	14/36	4/36	-6/36	0	14/36	12/36
5	19/36	8/36	-3/36	-14/36	0	16/36
6	24/36	12/36	0	-12/36	-16/36	0

Activity 7: Determine the optimal strategy for each player in the silent duel. For Player 1 this is the row with the maximum minimum value and for Player 2 the column with the minimum maximum value.

Conclusion: There are many variations of this simple model of a duel. For example, we could let the probabilities of the two duelists hitting their shots increase at different rates, or we could assume that they had more bullets in their pistols. Of course in real life, time does not increase in a discrete fashion and so the duelists could fire at any time between 0 and 6. According to David Blackwell, �[two-person duels] are the games for which the theory is clear and beautiful� (Albers & Alexanderson, 1985, p. 25). We hope that you have enjoyed these games and that you have developed an appreciation for David Blackwell�s work on the theory of duels.

Activity Sheet 2: David Blackwell and The Prisoner�s Dilemma

Women and Minorities in Mathematics

Incorporating Their Mathematical Achievements Into School Classrooms

Activity Sheet 1: David Blackwell and the Theory of Duels

Game 1: The Noisy Duel

Game 2: The Silent Duel

Mathematical Analysis of the Duel

Game 1: The Noisy Duel

Game 2: The Silent Duel

Activity Sheet 2: David Blackwell and The Prisoner�s Dilemma