Classroom Activities on Carolyn Gordon's Drums
Picture From
You Can't Always Hear the Shape of a Drum by
Barry Cipra
|
A mathematical drum is any shape in the plane that
has an interior and a boundary. The interior vibrates while the
boundary determines which frequencies are allowed.
Carolyn Gordon, David Webb and Scott Wolpert
found two mathematical drums that
produce exactly the same sound. When made into real drums,
with drumheads of the same material and tension, the two shapes
would resonate at exactly the same frequencies.
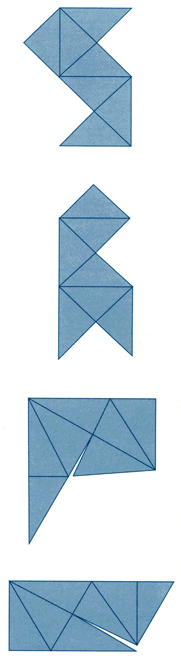
The Top Two Drums Sound Alike
1. Explain why these drums are considered to be shaped
differently.
2. Prove that they have the same perimeter. Explain your work.
3. To prove that they have the same area,
cut one of the drums into triangular pieces, along the
given lines, and then match up the pieces onto the first drum
to show that they have the same area. Did you get them to match up?
It is much harder to prove that the drums sound the same - a
mathematical proof is needed.
The Bottom Two Drums
4. Can you show that these drums have the same perimeter and area?
Explain how or why not.
|
Here are Carolyn Gordon and her husband David Webb holding the
drums that they found sounded the same.
Notice that their t-shirts contain the top two drums from
the picture above! The top drum is on our left on the shirt, and is
tilted. The 2nd drum from the top is on our right on the shirt,
and is also tilted.

1. Explain why the drums that they are
holding have different shapes.
2. Look at the figures
drum 2 and drum 1.
Notice that drum 2, which has the grey shading on a part of it,
is the drum that
David is holding, while drum 1 is the drum that Carolyn is
holding. Cut drum 2 and two copies of drum 1
out along the black edges.
Take one of Carolyn's drum (drum 1)
and cut it apart to try and fit it onto David's drum
to show that they have the same area.
Hint: First try folding Carolyn's drum so that you make five pieces,
two of which are crosses of the same size, and the other three half of
a cross (split along the diagonal of the square in the middle of the
cross)
Then cut along your folds and try to fit the pieces onto
David's drum.
Which piece doesn't fit nicely?
Sketch a copy of that piece here.
3. These drums sound the same so they must have the same
area and perimeter, and so something must be wrong with our
models. Explain what is wrong with the model of drum 1.
Hint: Match up the cross of your uncut drum 1 with the
cross that Carolyn is holding if front of David's shoulder.
Now look at the the edge of drum 1 which starts just below
David's wrist and runs up towards his elbow, and the edge
which starts at the same place, but heads straight down his
jeans leg. Compare your model with the model in the picture.

