Classroom Worksheet on Hypatia's Possible Work on Archimedes Dimension of the Circle

by Dr. Sarah

Hypatia 370?-415

Hypatia is the first woman mathematician about whom we have any biographical knowledge and knowledge of her mathematics. Hypatia translated works, added exercises and reworded concepts to make them easier to understand, and so she helped works to survive through many centuries. Hypatia was the first woman to have a profound impact on the survival of early thought in mathematics [1].

Since Hypatia lived so long ago, it is hard to know exactly what she worked on, although we do have some specific historical evidence of her mathematics [2, 4 and 6]. Wilbur Knorr, a math historian, has done a lot of research on Hypatia's possible mathematics [5]. He has identified a certain style of writing that he attributes to Hypatia. He learned new languages so that he could analyze different versions of Archimedes Dimension of the Circle in Hebrew, Arabic, Latin and Greek. He suggests that one of the versions shows Hypatia's influence. We'll look at the proof of Archimedes Dimension of the Circle, and in this way see some mathematics that Hypatia might have worked on.

Archimedes Dimension of the Circle

Statement: For any circle, one-half the perimeter times the radius is equal to the area.

Worksheet Activity: Using formulas for the area and perimeter (circumference) of a circle, in terms of the radius, show that the statement is true.

Proof: Archimedes and mathematicians who later translated his work, such as possibly Hypatia, were not working with these formulas, so they had to find another way to prove the statement. So, assume for contradiction that we have a circle with one-half the perimeter times the radius not equal to the area.

Let Z=1/2 * perimeter of the circle * radius of the circle. Then Z < area of the circle or Z >area of the circle.

Assume that Z < area of the polygon. By Euclid's Elements XII, we can inscribe a polygon in the circle so that the area of the polygon is bigger than Z.

Worksheet Activity: What happens to a polygon inscribed in a circle as the number of sides gets really large? Use this and the fact that Z < area of the circle to explain why it makes sense that we can inscribe a polygon in the circle so that the area is bigger than Z.

Let A be the center of the circle. Since the polygon is inscribed, then H is also the center of the polygon. Let B and C be adjacent vertices of the polygon so that BC is one of the sides, and let K be the midpoint of BC (see the picture for and example of this set up when the polygon has 5 sides). Now the perimeter of the polygon is less than the perimeter of the circle.

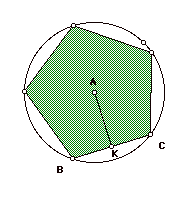
Worksheet Activity: Look at points B and C. Look at line BC, and also the circular arc extending from angle BAC that also joins B and C. Which is longer? Why? Use this to explain why the perimeter of the polygon is less than the perimeter of the circle.

Also, AK is less than the radius of the circle, since A is the center of the circle and K is inside the circle, while the radius of the circle extends from A to the edge of the circle, past K. Restating, we see that AK < radius of the circle, and perimeter of the polygon < perimeter of the circle. These are all positive numbers, so we can multiply and still preserve the less than sign. Therefore

AK * perimeter of the polygon < radius of the circle * perimeter of the circle.

Multiplying both sides by 1/2, which still preserves the less than sign, we see that

1/2 *AK * perimeter of the polygon < 1/2 *radius of the circle * perimeter of the circle = Z, by definition of Z.

Worksheet Activity: Connect points A and B, and A and C to form lines AB and AC. Then ABC forms a triangle. Why is this triangle isosceles? Why is AK perpendicular to BC? Why is 1/2 *AK *BC the area of this triangle? Use this to show that 1/2 * AK * perimeter of the polygon is equal to the area of the polygon.

Hence the area of the polygon = 1/2 *AK * perimeter of the polygon < Z, and so we have arrived at a contradiction to our assumption that the area of the polygon was bigger than Z.

Assume that Z > area of the circle. Then we can circumscribe a polygon about the circle so that the Z is greater than the area of the circumscribed polygon.

Worksheet Activity: Draw a picture, label points, and then use a proof similar to the one above (although a bit different) to arrive at the contradiction Z > Z. Then see the end of the proof on the next page.

Hence we have arrived at a contradiction to our assumption that a circle with one-half the perimeter times the radius not equal to the area. Therefore every circle has one-half the perimeter times the radius equal to the area, as desired.

References

1. Hypatia web page by Ginny Adair, Class of 1998 (Agnes Scott College), http://www.agnesscott.edu/lriddle/women/hypatia.htm

2. Hypatia's Mathematics: A Review of Recent Studies, by Edith Prentice Mendez

3. Hypatia's Work on Archimedes Dimension of the Circle web page by Dr. Sarah Greenwald, http://www.cs.appstate.edu/~sjg/womeninmath/circle.html

4. The Primary Souces for the Life and Work of Hypatia of Alexandria web page, by Michael A.B. Deakin, http://www.polyamory.org/~howard/Hypatia/primary-sources.html

5. Textual Studies in Ancient and Medieval Geometry by Wilbur Knorr

6. Women of Mathematics: A Biobibliographic Sourcebook by Grinstein and Campbell, p. 74 - 79.