The Life and Mathematics of David Blackwell

Of all the truly extraordinary African American mathematicians whose work began before the late 1960�s, David Blackwell is the most well known mathematician. He is considered, probably, the greatest black mathematician that has ever lived.

�David Harold Blackwell was born on April 24, 1919 in Centralia, Illinois, a town of 12,000 people located on the Mason-Dixson line.� (African Diaspora). As a young man growing up in Southern Illinois, Blackwell was lucky to attend a mixed school instead of the all black schools that were the norm in most areas of the nation at that time. Blackwell states, �Southern Illinois was probably fairly racist. But I was not even aware of these problems -- I had no sense of being discriminated against.� (AD) While going to school he was not interested in the more elementary math subjects such as algebra, trigonometry, and calculus. It wasn�t until he was a junior in high school that he found that he truly loved math. This came about after taking an introductory analysis course. �That was the first time I knew that serious mathematics was for me. It became clear that it was not simply a few things that I liked. The whole subject was just beautiful.� (AD)

After graduating from high school, Blackwell entered the University of Illinois in 1935. He received his A.B. in 1938, his A.B. in 1939 and his Ph.D. in 1941, all in the subject of mathematics. After graduation, he was appointed a Postdoctoral Fellow at the Institute for Advanced Study for a year. This position made him an official visiting fellow of Princeton University. This is where Blackwell first dealt with racism. The president of Princeton writes to the Institute for Advanced Study and admonishes them for allowing a black to become a Postdoctoral Fellow and that the Institute is abusing Princeton�s hospitality by allowing this to happen. But Blackwell was lucky. This was the time in American history when the attitudes towards blacks began to change. The 1950's and 1960's was when, with Brown vs. board of education and Martin Luther King; blacks began to see less persecution and began to receive more equal treatment. As most of the research says, Blackwell was lucky because through out most of his career he dealt very little with instructional racism and he was the first black that this happened to. This allowed him access to his future positions and accomplishments.

During his many years in the field of mathematics, Blackwell accomplished many outstanding achievements in international mathematics. �He was President of the Institute of Mathematical Statistics in 1955. He has also been Vice President of the American Statistical Association, the International Statistical Institute, and the American Mathematical Society and President of the Bernoulli Society. �In 1965 he became the first African American named to the National Academy of Sciences� (AD) He is an Honorary Fellow of the Royal Statistical Society and was awarded the von Neumann Theory Prize by the Operations Research Society of America and the Institute of Management Sciences in 1979. He is also a member of the American Academy of Arts and Sciences. He has received honorary degrees from the University of Illinois, Michigan State University, Southern Illinois University, and Carnegie-Mellon University.� (Conversation)

As the greatest black mathematician of his time, Blackwell was able to cross the race lines and succeed. He wrote over 50 papers and was allowed to succeed in the white mans world. Not only was he a great mathematician but also a winner when it came to the game of life.

David Blackwell wrote over 50 papers and two books containing such topics as game theory and Markov matrices. Blackwell was not interested in algebra or trigonometry while he was growing up but found geometry to be the most fascinating part of mathematics. He received his masters and doctorate in mathematics. He is a natural born teacher and loved working at Stanford University.

To understand what a Marko matrix is we must first define a probability vector. A probability vector has entries that are non-negative numbers and add to one. An example of a probability vector would be [.5, 0, 0, .5]. Square matrices whose columns are probability vectors are called stochastic matrices or Markov matrices. An example of a Markov matrix is as follows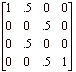. We will talk later about Markov chains.

Markov matrices can be used in predicting how things will pan out in the future. A perfect example would be to try and see how populations will turn out in the future. A scenario that we might be interested in would be to see how populations migrate within a country from urban to rural setting. For example (3) in a country with the current population we might be interested in seeing how populations migrate within a country from urban to rural settings.

For example in a particular country the current population has 45% living in urban areas and 55% living in rural areas. Each year 6% of the urban population moves to rural areas and 94% stay in the urban areas. Also each year 9% of the rural population moves to urban areas and 91% stay in the rural areas. We are now concerned with what will happen to the population in the future. A person may wonder if eventually everyone will live in urban areas or if the trend is to live in the rural areas. To figure this out we must form a matrix. The easiest way to make up the matrix is to write the equations that explain how the population moves each year. We will use u_k to represent the urban population in k years and r_k to represent the rural population in k year. The equation for the urban population is as follows

U_k+1= .94u_k+.09r_k.

This equation states that 94% of the original population plus 9% of the rural population is the new population after k years. The equation for the rural population is as follows

R_k+1= .06u_k+.91r_k

This equation states that 6% of the urban population plus 91% of the rural population is the new population of the rural area after k years. From these two equations we can form the following matrix . We will explain why this is the correct matrix in a moment.

To understand how these matrixes work we should review matrix multiplication. Using the following matrix we will show the process.

Looking at the previous matrix dealing with the population situation we can substitute u_k for c₁ and r_k for d₁ to get . Then applying the matrix multiplication we see we get the following equations.

I. A₁*u_k+a₂*r_k=u_k+1. From this equation you can see that .94 must be a₁ and .09 must be a₂

II. B₁+u_k+b₂*r_k=r_k+1. From this equation you can see that .06 must be b₁ and .91 must be b₂.

Now we should check to see what would happen if the columns were to be reversed. If we were to reverse the columns the matrix would look like . Now if we apply matrix multiplication we will get the following equation.

I. U_k+1=.09*u_k + .94*r_k which states that 9% of the urban population plus 94% of the rural population makes the new population for the urban areas after k years. This is an incorrect statement.

This shows the importance of having the columns in the correct order. Now we must check to be sure that this matrix is a Markov matrix. We must check to see that each column of the matrix adds up to one.

Now that we have established how to form the matrix we need to look into the original question of what will happen to the population in the future. If we take the Markov matrix and raise it to the number of years we are interested in then multiply it by the original distribution we will receive the new population. Like stated earlier the original population for the urban area was 45% and 55% for the rural area. The statement just explained looks like the following equation.

Now applying this formula we will look to see what happens after 10 years, 50 years, 100 years, and 150 years. We get the results by taking the Markov matrix and multiplying it by itself the number of years we are interested in. The results we get are as follows.

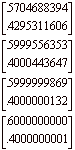
These matrices are what we call a Markov chain. In this example we see that the matrix is converging to . This matrix is called the steady-state vector. Not all Markov matrices will converge to a steady-state vector. The ones that do converge are considered �regular� stochastic matrices.

A Markov matrix is one topic that David Blackwell covers in his papers. He also likes to deal with probability and chance. In several of his papers Blackwell discusses game theory. This deals with likelihood and chance. The one paper we are going to take a look at focuses on a type of game called �The Big Match�.

In the paper �The Big Match� Blackwell deals with a two-person zero sum game. A game is an interaction or exchange between tow (or more) players, where each player attempts to make a choice (or �move�) towards the other layer in such a way that he could expect a maximum gain, depending on the other�s response. Zero-sum games are games where the amount of �winnable goods� is fixed. The other player therefore loses whatever the other player gains: the sum of gained (positive) and lost (negative) is zero.

In Blackwell�s paper he explains the following game. �Every day player 2 chooses a number,0 or 1, and player 1 tries to predict player 2�s choice, winning a point if he is correct. This continues as long as player 1 predicts a 0. But if he ever predicts a 1, all future choices for both players are required to be the same as that day�s choices; if he is wrong on that day, he wins zero every day thereafter.� This is a bit complicated but in simpler terms as long as player 2 keeps choosing 0, player 1 can pick either 0 or 1. Then as soon as player 2 picks 1 each player mist keep their choice of that day. This means that either player 2 guessed correctly and will now win everyday from now on or he guessed wrong and will now lose everyday.

Now we will go through a few situations of how the game might work out.

Example 1: Player 2- 0, 0, 1, 0, 1, 1, 0, 0, 1, 0, 0, 0, 0, 0, 0, 0, 0�

Player 1- 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 1, 1, 1, 1, 1, 1�

In this example we see that player 1 wins seven times before he chooses 1 and then he loses from there on out.

Example 2: Player 2- 0, 0, 0, 1, 1, 0, 1, 0, 1, 1, 1, 1�

Player 1- 0, 0, 0, 0, 0, 0, 0, 0, 1, 1, 1, 1�

In this example we see that player 1 wins five times before he chooses 1 and then he wins from there out.

There is a 50% chance that player 1 will guess the correct choice of player 2 since he is either going to guess right or wrong. . The best strategy for player 2 is to toss a fair coin every day. This strategy is the best because there is a 50 % chance that player 1 will guess his choice and so it only makes since to let a fair coin make the random choice. Unfortunately, player 1 has no optimal strategy. The only thing he might want to do is to continue picking 0 noting when he loses and wins and see if there is a probability of when it would be best for him to pick a 1. Blackwell has formed a formula to help player 1 decide when he should pick 1 or is he should never pick 1. With the following example I will walk you through this procedure. In the formula we will use an N, which is a large non-negative number. We will keep N an arbitrary number. We now need to find the excess of zeros over ones, at any given stage of the game, which is the same thing as the number of wins over losses for player 1. We find this excess because the game is still continuing so player 1 has chosen all 0�s up to this point. Lets assume that player 2 has picked 99 1�s and 1 0 and player 1 has only chosen 0�s up to this point. In this case it is 1-99=-98, which is equal to k100 or the excess of 1�s over 0�s. Then pick with the probability of P(k_n+N)=P(-98+N) where P(m)=1/(m+1)2. The final probability is P(-98+N)=1/((-98+N)+1)2. This is a very small denominator creating a large probability that if player 1 were to guess a 1 there is a great chance that he would be correct. Now there are two factors involved when looking at this probability. For one player 1 sees that there are 99 1�s and so he would expect that if player 2 is using a coin then a 0 should be coming soon. Therefore he would continue picking a 0. On the other hand, we do not know if player 2 is using a coin. It is possible that he is using another strategy to pick his number. The second factor involved is that player 1 has been losing a lot and therefore you would believe that player 1 should start winning and therefore would want to stay picking 0. This formula is only a strategy for player 1 to go by. It is important for player 1 to not pick 1 until they are certain that a 1 is coming next because if they guess a 1 and player 2 has chosen a 0 then player 1 will lose from there on out.

At the other extreme we can look at another example where player 2 chooses 99 0�s and 1 one. In this case K100 is 99-1=98. P(Kn+N)+P(98+N) where P(m)=1/((98+N)+1)2. This creates a large denominator making the probability very small probability. This probability states that if player 1 were to guess a one there is a small chance that he would be correct. Once again there are two factors that we must consider along with this probability. One factor is that player 2 has chosen 99 0�s and if he were using a coin then player 1 would expect a 1 to come along soon. Therefore player 1 would want to pick a 1. There is a possibility that player 2 is not using a coin and using some other method to pick his number. The other factor that we must take into consideration is that player 1 has been winning a lot and so he would think it would make since to start losing soon therefore leading him to want to pick a 1.

Markov matrices and game theory is used in society in many different ways. Markov matrices are used with populations like the example we went through. Also it is used with animal populations to see how various animals would coexist with each other. �Game theory develops general mathematical formulas and algorithms to identify optimal strategies and to predict the outcome of interactions.� Game theory is used in economics with the stock market, mathematics, statistics, political science, and psychology.