These are actual student papers that were not designed to be web pages. They may contain historical, grammatical, mathematical, or formatting errors. These papers were graded using the criterion mentioned in the paper directions, and the writing checklist. The test review sheets and the WebCT tests are good indicators of the mathematics that was discussed in class during and/or after each presentation.
Clarence Stephens

         Katy Rountree

                             Olivia Stanley

 

Clarence F. Stephens

           

 

During a time in history when African Americans were considered inferior to whites, few men of color were able to achieve educational success.  However, Clarence Stephens overcame many racial barriers to become only the ninth African American to earn a Ph.D. in mathematics.  In addition to being a superior mathematician, he has also been highly regarded as a pioneer of African Americans in mathematical education, as his methods of teaching are recognized as some of the �most profound in producing mathematics majors (MAD1).�  

Clarence Francis Stephens was born on July 24, 1917 in Gaffney, South Carolina (SC).  He was the fifth of six children, three girls and three boys.  His parents were Sam and Jeannette Morehead Stephens (MAA).  Sadly his mother died when he was two and his father died when he was eight years old (MAA).  All six children went to live with their grandmother who died two years after Stephens� father.  No relative could take in all six children, so the had to live with different relatives. 

Stephens lived with his great aunt Sarah in Harrisburg, North Carolina (NC), in a three-room house.  Two of the rooms were bedrooms, one of which was occupied by the two elementary school teachers.  The other room in the house was the kitchen, where all homework, dining, and socializing took place.  When Stephens became old enough to go to high school, he contemplated running away to go to school in the north, as there were no high schools in Harrisburg.  To avoid having her brother run away, Stephens� oldest sister Irene arranged for him to go to the Harbison Institute, a boarding school in Irmo, SC, provided that after she paid the first year�s tuition, he would pay the subsequent years� tuition.  Irene also made it possible for Stephens� brothers to go to Harbison Institute, with the same condition of tuition payment that Stephens had.  A job working on the Harbison farm in the summer paid for their winter schooling (DAAS).  Stephens also worked as a kitchen helper and dusted and swept the classrooms every day of the school year to supplement his room and board. 

An indication of Stephens� intelligence is shown by his placement test into Harbison.  He was thirteen years old and placed in the eighth grade alongside students who were over twenty (DAAS).  In addition, Stephens� teacher Dean Robert Boulware would have him present strategies for solving mathematical problems to the classroom.  Stephens would also tutor his classmates and help them with their math homework.

Stephens was very popular in high school.  He played football and was on the varsity baseball team.  Stephens was a well-rounded student.  During his school career, he was involved in various extracurricular activities.  He held the lead role in his senior play, was elected to class president his senior year, and was a good debater (DAAS).

Despite their rough childhood, all six Stephens children graduated college (MAA).  Stephens, his brothers, and one sister attended Johnson C. Smith University (JCSU) in Charlotte, NC.  Mathematics was the chosen field of study for all the boys in the family.  Stephens graduated JCSU in 1938 and then enrolled at the University of Michigan to begin his graduate studies in mathematics in the fall.  Stephens received his M.S. in 1939 and his Ph.D. in 1943 in mathematics from the University of Michigan (MAA).

While Stephens was attending school at JCSU, his only plans were to become a high school math teacher.  His decision to attend graduate school was seemingly impulsive.  The Dean of the College of Liberal Studies, T. E. McKinney, had returned from a visit to the University of Michigan, saw Stephens one day during his senior year at school, and said to him that the University of Michigan was where he needed to go.  So Stephens went to the University of Michigan for his master�s degree (MABPM).

Stephens had not considered getting his doctorate; he was just going for his master�s.  He had considered going to law school.  As a lawyer Stephens believed he could help the poor people he felt were always being exploited.  However, he could not become passionate about law, so he just figured he would teach high school math.  Like his decision to obtain his master�s degree, his decision to further his education with a doctorate degree was seemingly impulsive.  While talking to one of his professors, George Rainich, one day, Stephens was encouraged to return to school in the doctoral program.  Having a professor assume his return to further his education opened Stephens� eyes to his potential.  Professor Rainich�s comments were so inspiring that Stephens set more personal goals that he could achieve in the mathematical field.    

Stephens faced great difficulty in acquiring his graduate degrees.  During the time when Stephens was in school, he had to wait tables to pay for tuition, as there were no teaching assistant positions for African Americans (Stephens).  He also worked as a deliveryman for a local drug store that paid six dollars a week (DAAS).  In addition, Stephens served time in the Navy while working on his degrees.

After completing his tour of duty in the Navy, Stephens took a position as a professor of mathematics at Prairie View A. and M. College in Texas in 1946.  He spent little time in this position because he was given the opportunity to become the department chairman in the mathematics department of Morgan State University in Maryland.  After fifteen years at Morgan State, 1947-1962, Stephens again took on the role as a mathematics professor, but this time it was at the State University of New York (SUNY) at Geneseo.  He stayed at SUNY at Geneseo for seven years before going to the SUNY at Potsdam (MABPM pp. 63-65).

The SUNY at Potsdam was predominantly a training school for secondary mathematics education majors when Stephens arrived.  In addition, in 1969, there was no faculty in the math department who had received their Ph. D. from SUNY at Potsdam.  Furthermore, no student who had graduated with a B. S. in mathematics from Potsdam had gone on to earn a Ph. D. in mathematics.  Stephens believed this should be changed.  Thus, he devoted much time and effort into developing methods and strategies of teaching mathematics to encourage students to pursue advanced degrees in mathematics.  His methods were very successful in that at least eleven of his students went on to earn a Ph. D. in mathematics (MABPM). 

Because of his drive and ability to learn math and his desire to educate others in math, Stephens has received many honors and awards.  He received the Julius Rosenwald Fellowship in 1942.  In addition he received a Ford Fellowship and had the honor of being a member of the Institute for Advanced Study in Princeton, New Jersey from 1953-1954, working with such well-known people as Dr. Albert Einstein.  He received an honorary Doctorate of Science from JCSU in 1954.  In 1962, Stephens was honored for his many distinguished contributions to mathematical education by Governor J. Millard Tawes of Maryland, and again in 1987 by Governor Mario Cuomo of New York (NY) (MAA).  He received the SUNY Chancellor�s Award for Excellence in Teaching in 1976-1977 while he was working in Potsdam, NY. He was inducted and permanently placed in the National Museum of American History, Smithsonian Institute, as a part of the �A Living History Project on Black Americans In the Sciences� (MAD).  In the past eleven years he has received three more honorary doctorates: from the University of Chicago in 1990, from SUNY in 1996, and from Lincoln University in 2000 (MAA and MAD).        

Today Stephens lives with his wife Harriette on their farm in Conesus, NY.  His mathematical influenced is evident in that his daughter, H. Jeanette Stephens, and his son, Clarence F. Stephens Jr. both have advanced degrees in mathematics.  Even in his retirement Stephens is often asked to speak at colleges and universities in the United States and Canada about mathematics education (MAA). 

Dr. Stephens chose the topic of difference equations for his doctoral project.  His advisor thought this topic was too difficult for him, but the fact of the matter was, once Stephens got the problem into his head, it took only two weeks to complete his work (DAAS, p.299).  Perhaps Stephens� reason for choosing this topic was discussed in his paper, �Nonlinear Difference Equations Analytic in a Parameter.�  In this paper, Stephens quotes, �Up to the present time but little progress has been made in the development of a systematic theory of nonlinear difference equations from the point of view of general function theory (Stephens2, p. 268).�  The main purpose of this particular paper is to investigate the �solutions of nonlinear difference equations analytic in a parameter (Stephens2, p.268).� 

            A student of mathematics could better understand the nature of Stephens� work with a deeper knowledge of nonlinear difference equations.  These particular equations have been described as, �very complicated equations which, however, have many practical uses (DAAS, p. 299).�  Difference equations can be used to describe things such as changes of the texture of oil under the differing conditions of heat and pressure in an engine (DAAS, p. 299).  They also can be used to model certain interactions of individuals such as the spread of rumors and sicknesses, and natural growth such as the growth colonies in species populations (Bauldry).  Difference equations are used to model discrete data such as traffic patterns and temperature measurements.

            In order to understand a model of a difference equation, one must first understand the definition.  A difference equation is an equation written in terms of changes of a particular function. These functions, which take the form F(n, an+2, an+1,�,an), define a rule to transform whole numbers into elements of a sequence.

            The rule F, gives a linear equation if the terms are multiplied only by a constant. For example, the function Nt+1 � Nt = F � G, is a difference equation representing the traffic patterns on and off a particular highway. In the general form the traffic equation looks like F (t, Nt+1, Nt), where the rule F gives the form Nt+1 � Nt = F � G combined with one initial condition, makes the generated sequence unique. This equation is a linear equation because the terms, Nt and Nt+1, are multiplied only by constants. If the terms are multiplied by one another the equation is nonlinear. If F (n, an+1, an) takes the form of an�2 + an+1 = an, then it is nonlinear because an is a term and is multiplied by itself. The factors that make a difference equation nonlinear are powers, products, roots, or functions. Here is a difference equation that is nonlinear by all these factors: F (n, an+3, an+2, an+1, an) with the rule, �(an+3) * an+2! + tan (an+1) = an5 (Moorefield).

The graph of a linear difference equation is not necessarily a line. For example, some graphs have been known to be parabolas.  Stephens worked on nonlinear difference equations for his doctoral dissertation.  One can tell that these equations are nonlinear because their forms contain aspects of nonlinear equations mentioned above. 

An initial model of a nonlinear difference equation can be formed by using the potential spread of a rumor throughout a specific population.  To do this, one would need algebraic representations of time (n which represents one more than the actual number of time intervals passed), the number of people who know the rumor after n-1 time intervals (an), and the number of people who do not know the rumor.  One will obtain this representation by taking the total population (P) and subtracting the number of people who do not know the rumor (an).  The result is P � an equaling the number of people who do not know the rumor. 

            Based on this information, one can build the difference equation an = an-1 + c * an-1 * (P - an-1), where a1 = b.  Our values that remain constant are c, which is the constant of proportionality that governs the rate at which the rumor actually spreads, P, the population size, and b, which is the number of people who start the rumor.  This leaves an and n to be the variables.  Because the an-1 terms are multiplied together, the resulting degree of this difference equation will be two, thus making it nonlinear.

            Since the difference equation that is formed has three parameters, c, P, and b, it is necessary to estimate the values of these constants.  The values chosen in the book by Bauldry on page 27 imply that the population size should be 1.00 because a large population size is unlikely to affect the way a rumor spreads.  The initial value of b is taken to be seven percent of the population, and c is taken to be 0.05, which assumes the number of people who learn the rumor equals approximately five percent of the interaction between people who know and people who are unaware of the rumor (Bauldry, p. 27). 

            Substituting the indicated values for the appropriate parameters forms the new difference equation which is of the form an = an-1 + 0.05 * an-1 (1 - an-1).  The graph of this difference equation looks like the following:

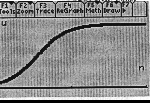
(Bauldry, p. 28).  This graph can be obtained by using a graphing calculator and plugging the equation into the �y =� menu.  In order to do this, one must substitute the variable �x� in for an-1.  Thus, the equation entered into the graphing calculator will be of the form y = x + 0.05 * x (1 � x).  The graph of this difference equation is nonlinear because the equation itself is nonlinear.  It is sometimes hard to tell the difference between a linear and a nonlinear difference equation just by examining their graphs.  In fact, it is necessary to look at the equations because parts of the graphs could look similar. 

These nonlinear difference equations are similar to the equations Stephens worked on, only these are much simpler.  The previous example provides just an introduction and overview of nonlinear difference equations.  Stephens wrote papers on �Difference equations having no linear terms in dependent variables and parameter (Stephens2, p.268).�  An example of a system of difference equations that he worked on in his dissertation is the system:  yi (x + 1) = fi (y1 (x), . . . , yn (x); P (x), x), fi (0, . . ., 0; 0; x) = 0 and (i = 1, . . . , n), where P(x) represents a parameter that is independent of yi(x), and (i = 1,  . . . , n) is periodic of period one with respect to x (Stephens2, p. 268).  He formulated properties of these equations and proved their convergence.  Stephens displayed an in-depth knowledge of such equations as he published two papers based on nonlinear difference equations.

Clarence Francis Stephens is a very intelligent and dedicated man.  He had to surpass many racial obstacles and unfortunate circumstances to become the ninth African American to receive a Ph.D. in mathematics.  Stephens� love for mathematics developed at a very young age and he has devoted most of his life to the field.  He was once quoted as saying, �More than fifty years ago I came to the conclusion that every college student who desired to learn mathematics could do so.  I spent my entire professional life believing this was the case (MAD1).�  His many awards and honors in mathematics further prove that Stephens is truly a great African American pioneer in the field of mathematics.

 

 

 

 

References

 

Bauldry, Ellis, Fiedler, Giordano, Judson, Lodi, Vitray, West. 1997. Calculus: 

            Mathematics & Modeling. Addison Wesley Longman, Inc., Reading

            Massachusetts. (Denoted Bauldry in paper) Used in math part of paper to

            obtain rumor example of nonlinear difference equation.

 

Datta, Dilip.  Math Education at its Best: The Potsdam Model. Rhode Island

            University Press, Kingston, RI, pp 61-65. 1993. (MABPM) Good source for

            his teaching methods.

 

Discussion with Dorothy Moorefield.  (Moorefield) Very helpful with the math.

 

Distinguished African American Scientists of the 20th Century. �Clarence

            Stephens.�  P.296-301. (DAAS) The best source for biographical,

            educational, and career highlights.

 

http://www.maa,org/summa/archive/Stephn_c.htm (MAA) Good overview of life

            and work.

 

http://www.math.buffalo.edu/mad/morgan-potsdam_model.html (MAD2) Included

            repeated information.

 

   http://www.math.buffalo.edu/mad/PEEPS/stephens_clarencef.html (MAD1)

               Excellent biographical overview of Stephens� life and work.

 

http://www.potsdam.edu/MATH/quotes.html (POTSDAM) Contained

            quotes from Stephens.

 

Interview with Clarence Stephens on February 21, 2001.  (Stephens) Provided

            an all around good basis of information.

 

Nonlinear difference equations containing a parameter, Proc. Amer. Math.

            Soc.1 (1950), 276-281. (Stephens3) Used to examine Stephens� work on

            difference equations.

 

Nonlinear difference equations analytic in a parameter, Trans. Amer. Math.

            Soc.64 (1948), 268-282.  (Stephens2) Also used to examine Stephens�

            work on difference equations.