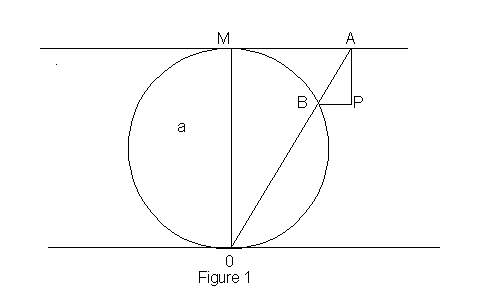
 The construction of the curve begins, in Figure 1 above, with a circle with diameter
The construction of the curve begins, in Figure 1 above, with a circle with diameter Very little is known about many early women mathematicians. Many women experienced extreme prejudices because of their sex, therefore limiting their success and acknowledgements in many fields such as mathematics. It is very rare for much to be known with certainty about beginning female mathematicians and almost impossible to find any original work. Maria Agnesi is a pioneering female mathematician that has a history with many aspects that can be labeled as accurate, and her book Analytical Institutions is the first surviving mathematical work written by a woman (Gray and Malakyan, 258-259).
Maria Gaetana Agnesi was born on May 16, 1718 in Milan, Italy. Maria was born into a rather wealthy family and was fortunate enough to receive an extensive education. Maria's father, Pietro Agnesi, was a strong advocate for the education of his daughter (Anges Scott 1; Smith 109). Whether or not he was a wealthy silk merchant or a professor at the University of Bologna are issues that are still debated. C.Truesdell states in an article that Anzoletti "effectively disposes of the tenacious old legend" that Agnesi’s father was a professor of mathematics (p 115), whereas Gray and Malakyan state that her father "occupied the chair of mathematics at the University of Bologna" (p 256).
It was very rare for a woman to receive an education during the eighteenth century, much less one as extensive as Maria's was. Maria's father provided her with highly respected tutors such as Don Ramiro Rapinelli, which happened to be a professor of mathematics at the University of Pavia. Maria demonstrated extreme intelligence at an early age (Alic 196), and became well versed in languages, philosophy, and mathematics
(Agnes Scott 1; Smith 109). At the age on nine she composed a Latin essay in favor of the higher education for women and it was successfully published when she was eleven (Gray 265). It is no surprise that with her incredible intelligence coupled with such high quality tutors that Maria became one of "the most celebrated Italian women in the scientific revolution" (Alic 196).Maria published a work called Propositiones Philosophicae in 1738, at the age of twenty. This work concentrated on philosophy and the natural sciences. Maria's father enjoyed inviting people that were considered important among society to his house so that they could converse with Maria and listen to how eloquently she could express her ideas and opinions. On one occasion Countess Clelia Borromeo, learned in languages, philosophy, natural sciences and mathematics, states: "…Signora Agnesi, twenty years of age, who is a walking dictionary of all languages, … I least desire to go; she knows too much for me" (Truesdell 116-7). Maria was not very fond of these visits and really did not enjoy her intelligence being demonstrated in front of others. Maria began to express an interest in becoming a nun, but the idea of her devoting her life in such a way was absolutely refuted by her father. Succumbing to her father's protests, Maria did not become a nun but devoted lots of her time to the study of religious books and mathematics (Agnes Scott 1-2).
While studying mathematics, Maria began to write about the works of other mathematicians. Maria wrote a commentary concerning one of L'Hopital's works on conic sections (Agnes Scott 1). After the death of her mother, being the oldest of 21 children, Maria took her mother’s place as care taker of the household. She became a tutor for her brothers and in an attempt to explain mathematics, she wrote Analytical Institutions (Gray 266). Maria oversaw the printing of her book, and Analytical Institutions was completely published in 1748. This two volume book was "a systematic text of algebra, analytic geometry, and calculus" (Kennedy 480). In the first volume, the material is in order on increasing difficulty, and the second volume treats ‘"infinitely small quantities"’ and is divided into three different sections. In order, the sections explain differential calculus, integral calculus, and antiderivative methods. In the end of the book she introduces fundamental differential equations. "She uses Leibniz’s continental language of differentials and infinitesimals rather than the fluxions of Newton"(Grey 262-3). After the book's publication, it began to receive wonderful reviews among many academics. One example of the book's praise was when "Pope Benedict XIV wrote to Agnesi saying that he had studied mathematics when he was young and could see that her work would bring credit to Italy and to the Academy of Bologna" (Agnes Scott 3). He appointed her an honorary lecturer at the University of Bologna, a position that she may or may not have accepted (Gray 267). If Maria did not accept the position as honorary lecturer, then it has been said that she lived the last part of her life helping others less fortunate than herself (Agnes Scott 3).
Within Analytical Institutions
, Maria discusses a certain curve that became known as the "Witch of Agnesi." The word witch became associated with the curve because John Colson, a Cambridge Lucasian Professor of Mathematics, mistook the word averseria (from Latin vertere meaning to turn) for the Italian aversiera (witch) when he translated the work into English in 1801 (Gray 258). The construction of the curve begins, in Figure 1 above, with a circle with diameter
The construction of the curve begins, in Figure 1 above, with a circle with diameter ![]() that is drawn tangent to the x-axis. The line M above the x-axis is also tangent to the circle and parallel to the x-axis. Line OA intersects the circle at the point B. Triangle APB is formed when a ray beginning at A and extended down intersects a ray that begins at B and extended to the right. The intersection of these two rays is P. Segment AP is parallel to diameter a and segment BP is parallel to the x-axis, thus triangle APB is drawn as a right triangle. To begin generating the curve, let point A move to the right. As point A moves, as shown in Figure 2, more and more right triangles are created. The connection of the vertices of the right triangles, for example point P is a vertex in the right triangle APB, actually forms the curve.
that is drawn tangent to the x-axis. The line M above the x-axis is also tangent to the circle and parallel to the x-axis. Line OA intersects the circle at the point B. Triangle APB is formed when a ray beginning at A and extended down intersects a ray that begins at B and extended to the right. The intersection of these two rays is P. Segment AP is parallel to diameter a and segment BP is parallel to the x-axis, thus triangle APB is drawn as a right triangle. To begin generating the curve, let point A move to the right. As point A moves, as shown in Figure 2, more and more right triangles are created. The connection of the vertices of the right triangles, for example point P is a vertex in the right triangle APB, actually forms the curve.
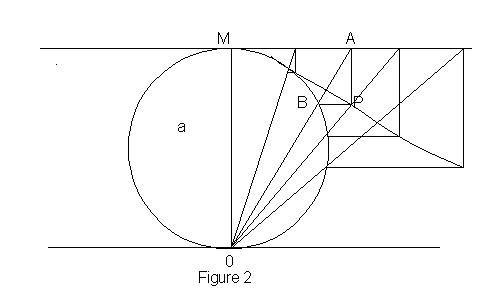 The curve can also be generated to the left of the circle as well as the right, which is shown in Figure 4 after the development of the equation for the curve, by following the same procedure as above.
The curve can also be generated to the left of the circle as well as the right, which is shown in Figure 4 after the development of the equation for the curve, by following the same procedure as above.
The equation of the curve is developed by first finding the relationship between x and y in Figure 3. In Figure 3, a segment starting at point A and containing the segment AP is extended all the way to the x-axis. A segment BK is also extended to the x-axis from point B. Point P is assigned coordinates x and y, and point B is assigned coordinates u and v. There is also a third segment, NP, that begins on diameter a and intersects the segment containing segment AP. Right triangles AMO and ABM share a common acute angle and are therefore similar triangles. Right triangles AMO and MBO are also similar
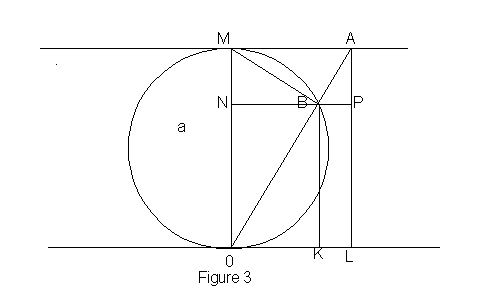 because they share a common acute angle, and triangles AMO and BPA are similar as well. Since triangles AMO and BPA are similar,
because they share a common acute angle, and triangles AMO and BPA are similar as well. Since triangles AMO and BPA are similar, ![]() . Another way of representing these proportions is
. Another way of representing these proportions is ![]() where x is the distance from point M to point A, and
where x is the distance from point M to point A, and ![]() is the distance from M to A minus the distance from point N to point B. Similarly,
is the distance from M to A minus the distance from point N to point B. Similarly, ![]() is the distance from point A to point L, and
is the distance from point A to point L, and ![]() is the distance from A to L minus the distance from point P to point L. By cross multiplying the proportion, one gets
is the distance from A to L minus the distance from point P to point L. By cross multiplying the proportion, one gets ![]() By distributing the
By distributing the ![]() and the
and the ![]() and solving for
and solving for ![]() it is found that
it is found that ![]() . By using the Pythagorean Theorem, in triangle OBK,
. By using the Pythagorean Theorem, in triangle OBK, ![]() where
where ![]() is the distance from point B to point K and
is the distance from point B to point K and ![]() is the distance from point O to point K. In triangle MNB,
is the distance from point O to point K. In triangle MNB, ![]() where
where ![]() is the diameter a minus the segment NO and
is the diameter a minus the segment NO and ![]() is the distance from point N to point B; in triangle QOB
is the distance from point N to point B; in triangle QOB ![]() . By using substitution,
. By using substitution, ![]() and this reduces to
and this reduces to ![]() Since
Since ![]() ,
, ![]() becomes
becomes ![]() . When one solves for y, the equation
. When one solves for y, the equation ![]() is found for the curve where
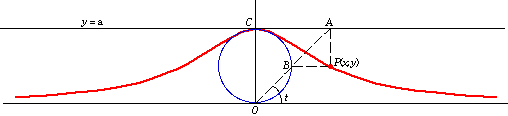
is found for the curve where ![]() can be various diameters for a circle (Gray and Malakyan, 259-260). The curve is useful in applications in physics that deal with the approximation of the "spectral energy distribution of x-ray lines and optical lines." (Spencer 415).
can be various diameters for a circle (Gray and Malakyan, 259-260). The curve is useful in applications in physics that deal with the approximation of the "spectral energy distribution of x-ray lines and optical lines." (Spencer 415).
Maria Agnesi did not become enthralled in the recognition that she received because of her book. Maria completed devoted her life to charitable work and theology after the death of her father (Grinstein and Campbell 2). Maria's book was revered among academics, and she became well respected by many because of the intelligence demonstrated in writing it. Maria was an exception to many rules because she was a woman that not only received a good education, but her writings concerning mathematics were published despite the fact she was a woman. Maria was surely an inspiration to other mathematicians, both male and female, and proof that women can do and understand mathematics. (
www.agnesscott.edu/iriddle/women/witch.html)