Dr. Sarah's Homer Part 2 for INTERNET EXPLORER

Homer
Changing Dimensions
from Treehouse of Horror VI 3F04
(10/30/95)
In Homer Part 1 we explored Homer's transition.
Yet, in most respects, the Simpsons already represent a 3-d world, while
an amoeba confined to a thin layer of water on a slide in biology class,
seems to more
accurately represent a 2-d world.
All of the Simpson's actions
indicate that they have use of three full dimensions
for movement (for example, drawing on a blackboard requires 3 dimensions,
and so does the way they hold out their arms, talk to each other, ...).
In addition, they use perspective viewing of objects.
We know that experts think that the universe has many more physical
dimensions than we directly experience. In order to try and understand
the idea of more physical dimensions, we will step back and try to
address an easier, but related question: How could a 2-d creature
understand the 3rd dimension?
In the process, we will see some ideas that at first appear counterintuitive.
We will look at 3-d objects in new ways, in an attempt to develop
visualization skills that we will need in order to understand the 4th
physical dimension. Next week we will revisit the intuition and ideas
we have learned within the context of the 4th dimension, and will look
at the practical utility of these abstract notions.
So, for now, assume that the Simpson's really were 2-d creatures living
in an x-y plane of some blackboard, as Dr. Frink suggests,
and that Homer and Bart had made the transformation to 3-d creatures.
While a 2-d Marge can't
really understand the 3rd dimension and would feel like there isn't any
room for another dimension, she could
see weird behavior occurring that suggests
that the 3rd dimension exists (for example the "wall" that Homer
disappeared into could not be explained using only 2 dimensions).
2-d Marge wouldn't be able to comprehend the concept of
depth or an entire 3-d Homer, since only 2-d pieces would make sense to her.
In the process, we will continue to explore the relationships between
geometry, visualization, art, history, philosophy and physics.
Taken from Hyperspace & A
Theory of Everything, by Dr. Michio Kaku
Taken from David Henderson's Experiencing Geometry in the
Euclidean, Spherical, and Hyperbolic Spaces
NAMES__________________________________________________________________
Work with one other person and
turn in one lab per group.
For each of the following questions,
read through carefully, watch the movies, read again, and drag through the
movie in
slow motion using your mouse.
THEN, ANSWER EACH QUESTION WITH BOTH PICTURES AND WORDS:
In
Davide Cervone's "Movie Slices of a Cube Passing Through Flatland"
(the blackboard),
pretend that 2-d Marge is standing in the middle of the
right side of the gray shaded
square (far enough to the right that the cube never whacks into her!).
Notice that Marge's view of something passing through near her
will be very different from your view of what actually passes through her
plane since she will not be able to see outside of her plane, and she
will only be able to see a part of her plane (think about standing outside
a building - you can't see all 4 sides at once). Answer questions 1-4 using
these ideas.
- What do we see in Marge's plane
when the first cube passes through her blackboard?
- What would she see when the first cube passes through her blackboard?
- What do we see in Marge's plane
when the second cube passes through her blackboard?
- What would she
see when the second cube passes through her blackboard?
- Use
Davide Cervone's "Dimensional Connections"
to explain how a square can be formed from a line.
- Search in this
text transcript of 3-d Homer segment and Did You Notice? by James A. Cherry
for Professor Frink's description of how a cube can be formed from
a square, and copy that down here.
-
Davide Cervone's "Cube and Hypercube Movies".
Read the text to the right of the first movie. Then watch the first movie
and answer the following question:
If 2-d Marge was standing at the bottom of the movie (near to the
controls), then what would she see (as a shadow in her plane of
existence) as the cube was formed from a square?
(I find it best to answer this by repeating the shadow part of the
movie.)
- There is an alternative way of forming a cube. Recall that we can form a
cylinder by taking a piece of paper and gluing the left and right sides
together. I could explain this to 2-d Marge by drawing a square and then
giving the gluing instructions as follows:
 . While she wouldn't understand how there could
be space to accomplish this feat, (since we need a 3rd dimension to actually
glue the edges together to form the cylinder) she could still
comprehend the gluing
instructions.
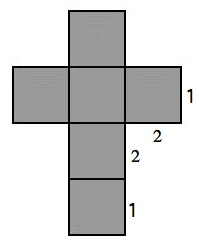
Take a look at Davide Cervone's "A Cube Unfolded"
Explain which sides you would glue together in order to form a cube
by labeling the gluing instructions on the figure below. (Hint: You will
need to glue 7 sets of edges together, so you may want to give the
instructions by labeling a set of 1s to be glued together, a set of 2s,
and so on...)
. While she wouldn't understand how there could
be space to accomplish this feat, (since we need a 3rd dimension to actually
glue the edges together to form the cylinder) she could still
comprehend the gluing
instructions.
Take a look at Davide Cervone's "A Cube Unfolded"
Explain which sides you would glue together in order to form a cube
by labeling the gluing instructions on the figure below. (Hint: You will
need to glue 7 sets of edges together, so you may want to give the
instructions by labeling a set of 1s to be glued together, a set of 2s,
and so on...)
- Go through the following movies to further help you develop
visualization skills.
Davide Cervone's "Perspective and Orthographic
Views of a Cube"
Davide Cervone's "Shadows of a Cube Rotating Above a Plane
Some other questions
- Why couldn't Marge eat if her mouth was located in the middle of
her head?
- Where would 2-d Marge's eyes have to be located for her to see things
in her plane of existence? Why?
- How could 2-d Marge and 2-d Lisa pass each other?
(Hint - think of them moving around in their 2-d plane-without-depth.)
|
|

 . While she wouldn't understand how there could
be space to accomplish this feat, (since we need a 3rd dimension to actually
glue the edges together to form the cylinder) she could still
comprehend the gluing
instructions.
Take a look at Davide Cervone's "A Cube Unfolded"
Explain which sides you would glue together in order to form a cube
by labeling the gluing instructions on the figure below. (Hint: You will
need to glue 7 sets of edges together, so you may want to give the
instructions by labeling a set of 1s to be glued together, a set of 2s,
and so on...)
. While she wouldn't understand how there could
be space to accomplish this feat, (since we need a 3rd dimension to actually
glue the edges together to form the cylinder) she could still
comprehend the gluing
instructions.
Take a look at Davide Cervone's "A Cube Unfolded"
Explain which sides you would glue together in order to form a cube
by labeling the gluing instructions on the figure below. (Hint: You will
need to glue 7 sets of edges together, so you may want to give the
instructions by labeling a set of 1s to be glued together, a set of 2s,
and so on...)
