Euclid's Book 1 Proposition 11: To draw a straight line at right angles to a given straight line from a given point on it.

Tools

Arrowhead      
Point              
Compass          
Straight Edge      
Alphabet      
Script View      

Sketchpad Construction

  • Choose the Straight Edge Tool and create a segment.
  • Choose the Alphabet Tool and label the endpoints of the line A and B.
  • Choose the Point Tool and create a point on AB.
  • Choose the Alphabet Tool and label this point as C.
  • Choose the Point Tool and create a point that is located on whichever is shorter from among AC or BC. AC or BC.
  • Choose the Alphabet Tool and label this point as D.
  • Choose the Compass Tool, click on C, drag the circle with your mouse and then click so that D lies on the edge of the circle centered at C.
  • Use the Point Tool to click down on the intersection points of this circle with the segment that is longer from among AC or BC.
  • Use the Alphabet Tool to label this intersection point as E.
  • Construct an equilateral triangle on DE as we did when we proved proposition 1 by constructing the 2 relevant circles whose intersection will create the 3rd vertex of the triangle.
  • Use the Point Tool to create the intersection point of these circles.
  • Use the Alphabet Tool to label this point as F.
  • Use the Straight Edge Tool to connect CF.
  • Measure Angle FCD by selecting the points in order using the Arrowhead Tool and the shift key and then using the Measure option.
  • Move points A, B, and D around in order to see that the angle measure stays the same.
    Notes:
              To de-select an object, choose the Arrowhead Tool and click the white background until the object is no longer highlighted.
              To save your work, under File, release on Save As... and save the file as anyname.gsp (for geometer's sketchpad).
              To create a script view of your work, select all of your work so that it is highlighted via Edit, Select All, and then choose the Script View Tool and release on Create New Tool. Check the Show Script View box and hit ok. To print a script view, Right-click (Windows) or Ctrl-click (Macintosh) on any object in the script, and choose Print Script View from the Context menu that appears.

    Proof of Prop 11 - Read this along with Appendix A (p. 287-292) of Sibley

    Given segment AB, and point C on it, we will prove that we can draw a straight line at right angles to a given straight line from a given point on it.
                                 
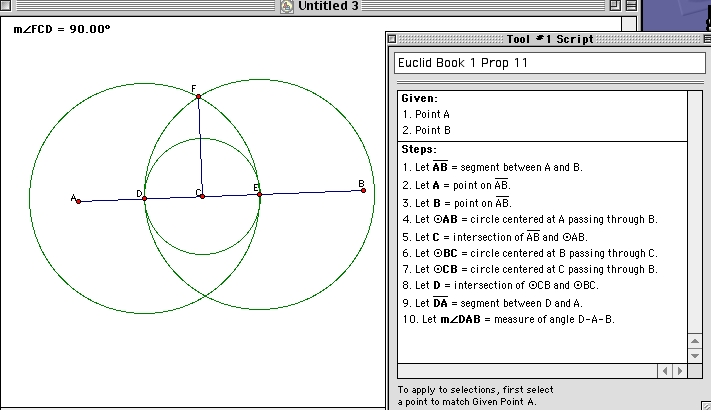
    Choose point D on the shorter of AC or BC. Common Notion 5 along with the implicit assumption that points exist on lines via Definitions 2, 3 and 4.
    Construct new point E on AB so that CE is equal to CD. Proposition 3, Definitions 17 and 4.
    Construct F so that F is the vertex of the equilateral triangle FDE on DE. Proposition 1
    Construct FC, DF and EF. Postulate 1
    Notice that DF=EF. Definition 20
    Therefore angle DCF equals angle ECF. Proposition 8
    Hence DCF and ECF are right. Definition 10
    Therefore the straight line CF has been drawn at right angles to the given straight line AB from the given point C on it, as desired.